July 29, 2015, 8:00am—5:20pm
Room 1405, Renmin University of China
|
Morning |
|
Afternoon |
|
Chair: Bei Hu |
Chair: Zejia Wang |
|
|
8:00-8:30, Steve Cantrell |
1:30-2:00, Yingdong Liu |
|
|
Break |
|
|
|
Chair: Steve Cantrell |
Chair: Yuan Lou |
|
|
10:10-10:40, Litao Han |
3:30-4:00, Yifu Wang |
|
|
Lunch Break |
|
Organizers: Steve Cantrell (University of Miami & RUC), Bei Hu (University of Notre Dame & RUC), Yuan Lou (RUC & Ohio State), Zejia Wang (Jianxi Normal University)
Host: Institute for Mathematical Sciences, RUC
Title and abstract
Resident-Invader Dynamics in Infinite Dimensional Dynamical Systems
Steve Cantrell
University of Miami
Abstract: We discuss an extension of the resident-invader dynamics for similar strategies from adaptive dynamics to infinite dimensional contexts, including that of reaction-diffusion equations. This is joint work with Chris Cosner and King-Leung Lam.
The reduction principle, the ideal free distribution,
and theevolution of dispersal strategies
Chris Cosner
University of Miami
Abstract: The problem of understanding the evolution of dispersal hasattracted much attention from mathematicians and biologists in recentyears. For reaction-diffusion models and their nonlocal and discreteanalogues, in environments that vary in space but not in time, thestrategy of not dispersing at all is often convergence stable within inmany classes of strategies. This is related to a “reduction principle”which states that that in general dispersal reduces population growthrates. However, when the class of feasible strategies includes strategiesthat generate an ideal free population distribution at equilibrium (allindividuals have equal fitness, with no net movement), such strategies areknown to be evolutionarily stable in various cases. Much of the work inthis area involves using ideas from dynamical systems theory and partialdifferential equations to analyze pairwise invasibility problems, whichare motivated by ideas from adaptive dynamics and ultimately game theory. The talk will describe some past results and current work on these topics
Strong Allee Effect in a Diusive Predator-prey System with a Protection Zone
Renhao Cui
Renmin University of China
Abstract: In this talk we consider that a reaction diusion predator prey system with strong
Allee effect and a protection zone for the prey. Dynamics and steady state solutions of the
system are analyzed. In particular it is shown that the overexploitation phenomenon can beavoided if the Allee effect threshold is low and the protection zone is large.
Computational design of protein structure, function and interaction
Xinqi Gong （龚新奇）
Institute for Mathematical Sciences，Renmin University of China
Abstract. Proteins perform specific biological functions with specific 3D structures, and usually interact with other partners or change their conformations to show different functions. But in many circumstances, it’s difficult and expensive for experiments to determine a protein’s basic information of structure, function or interaction. Here I will show how our computational algorithms help to design protein structure, function and interaction. Further importantly, biological experiments validate the power of our algorithms in three examples.
Evaluating the Impact of Test-and-Treat on the HIV Epidemic among MSM in China Using a Mathematical Model
Litao Han
Renmin University of China
Abstract:Background: Various studies have modeled the impact of test-and-treat policies on the HIV epidemics worldwide. However, few modeling studies have taken into account China’s context. To understand the potential effect of test-and-treat on the HIV epidemic among MSM in China, we developed a mathematical model to evaluate the impact of the strategy. Method: Based on the natural history of the CD4 count of people living with HIV (PLHIV), we constructed a dynamic compartmental model of HIV transmission among Chinese MSM to project the number of new HIV infection and prevalence over 10 years. We predicted the annual number of new HIV infections and the total number of MSM living with HIV and AIDS (based on Beijing data) between 2010 and 2022 under the following conditions: (1) current practice (testing rate of 50% and ART coverage of 39%); (2) both testing rate and ART coverage increasing to 70% in 2013; (3) both testing rate and ART coverage increasing to 90% in 2013; and (4) testing rate and ART coverage increasing by 5% each year until 90% since 2013. Results: Based on our model, if HIV test-and-treat policy was implemented among Chinese MSM, the total number of new HIV infections over 10 years (2013-2022) would be reduced by 50.6-70.9% compared with the current practice. When ART coverage for PLHIV increases to 57.5%, the turning point would occur on the curve of HIV new infections by 2015. A 25% reduction in annual number of new HIV infections by 2015 may be achieved if the testing rate increases from 50% to 70% and treatment coverage for PLHIV increases to 55%. Conclusion: Implementation of the test-and-treat strategy may significantly reduce new HIV infections among MSM in China. Great efforts need to be made to scale up HIV testing and ART coverage among Chinese MSM.
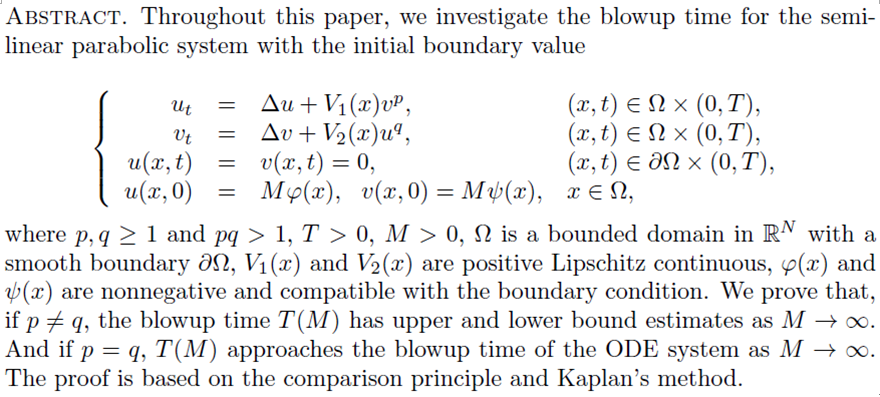
Upper bound of blowup time for a semilinear parabolic system
with potentials and large initial data
Yaodan Huang
Xian Jiaotong University
Modeling HIV-1 virus dynamics with both cell free virus infection
and cell-to-cell transmission
Xiulan Lai
Renmin University of China
Abstract：In this talk, we propose a mathematical model toconsider these two modes of viral infection and spread, direct cell-to-cell transfer of HIV-1 and virus-to-cell infection, in which infection age is also incorporated. Bya rigorous analysis of the model, we show that the model demonstrates a global threshold dynamics,fully described by the basic reproduction number, which is identified explicitly. The formula for thebasic reproduction number of our model reveals that the basic reproduction number of a model thatneglects either the cell-to-cell spread or
virus-to-cell infection might be under-evaluated.
On Global Dynamics of Competitive Systems in Ordered Banach Spaces
King-Yueng Lam
Ohio State University
Abstract: A well-known result in [Hsu-Smith-Waltman, Trans. AMS (1996)] states that in a competitive semiflow defined on the product of two cones in respective Banach spaces, one of the following outcomes is possible for the two competitors: either there is at least one stable coexistence steady state, or else one of the exclusion states attracts all trajectories initiating in the order interval bounded by the two exclusion states. However, none of the exclusion states can be globally asymptotically stable if we broaden our scope to the entire positive cone. In this talk, we discuss two sufficient conditions that guarantee, in the absence of coexistence steady states, the global asymptotic stability of one of the exclusions states. Our results complement the counter example mentioned in the above paper and are frequently applicable in
practice. This is joint work with Dan Munther (Cleveland State).
一类SEIS传染病模型的平衡解
Yingdong Liu
Beijing Jiaotong University
Abstract:利用分支理论证明了一类SEIS传染病模型的非常数平衡解的存在性，并计算了分支方向，由此刻画了此类传染病模型的门槛现象。
Laplacian on fractals
Tianjia Ni
Renmin University of China
Abstract: On Euclidean space, diffusion of heat is described by the heat equation. If the heat transport is undertaken in some disordered media such as porous rocks, the heat equation on Euclidean space is not suitable any more. Fractal is considered as an appropriate model for geometrical structure of most disordered media. However, the differential operator Laplacian, which is used in heat equation, on fractal can not be easily defined as on Euclidean space, since fractals may not have smooth structures. In this talk, we will define the Laplacian on a particular class of fractals. The main tool is the generalized Kakutani fixed point theorem.
Global classical solutions to vacuum free boundary problem of full Navier-Stokes equations with large initial data
Yaobin Ou
Renmin University of China
Abstract: In this talk, I`ll present a recent result on the the free boundary problem of Navier-Stokes equations for viscous ideal polytropic and heat-conducting fluids, when the density connects to the vacuum continuously. The global existence of classical solution to the problem with large initial data was established in this work.
Blow-up and Asymptotic Behavior of the Reaction-diffusion Equations
with Free Boundaries
Ningkui Sun
Tongji University
Abstract: We study the reaction-diffusion equations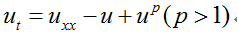
in a varying domain 
, whereg(t) and h(t) are two free boundaries.
We obtain the blowup-transition-vanishing trichotomy result. When blow-up
happens, we deduce that the blow-up set is a compact subset of initial domain
and the two free boundaries keep bounded.
Boundedness in a chemotaxis--haptotaxis model
with remodeling of non-diffusible attractant
Yifu Wang
Beijing Institute of Science and Technology
Abstract: This paper is concerned with the cancer invasion model
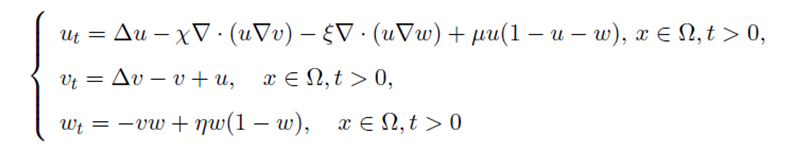
in a bounded smooth domain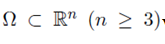
with zero-flux boundary conditions, where
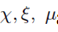and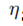 
are positive parameters. As compared to previous mathematical studies, thenovelty here consists of allowing for positive values of 
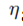, reflectingprocesses of self-remodelingof the extracellular matrix. It is shown that under appropriate regularity assumption on theinitial data 
, the corresponding initial-boundary problem possesses a unique classicalsolution which is global in time and bounded. This paper develops some Lp-estimate techniquesfor the full model of
Chaplain and Lolas.
Travelling Wave for Credit Rating Migration Problem
Yuan Wu
Tongji University
Abstract:In this paper, a free boundary model for pricing a corporate bondwith credit rating migration is proposed. The existence, uniqueness andregularity of the solution for the model are obtained. With reasonable assumptions, traveling wave solution is obtained, and the original problem is convergent to the traveling wave
solution.
Bifurcation for a free boundary problem modeling tumor growth with inhibitors
Zejia Wang
Jiangxi Normal University
Abstract：In this talk, we deal with a free boundary problem modeling tumor growth with inhibitors. This problem has a unique radially symmetric stationary solution with radius $r=R_s$. The tumor aggressiveness is modeled by a positive tumor aggressiveness parameter $\mu$. It is shown that there exist a positive integer $m^{**}\in\mathbb R$ and a sequence of $\mu_m$, such that for each $\mu_m(m>m^{**})$, symmetry-breaking solutions bifurcate from the radially symmetric stationary solutions.
On a class of Keller-Segel chemotaxis systems with cross-diffusion
Tian Xiang
Renmin University of China
Abstract：We study a class of Keller–Segel chemotaxis systems with cross-diffusion. By using the entropy dissipation method and assuming mainly the chemotactic sensitivity separates the cell density and the chemical signal, we first establish the existence of global weak solutions with the effects of cross diffusion included in ≤3-D. Then we show there is a critical cross diffusion rate δc such that no patterns may be expected for δ≥δc, while patterns are formed for δ<δc and their stability is also derived. In particular, in 1-D, patterns are always formed whenever δ<δcand the chemotactic coefficient is larger than an expressible bifurcation value, and there is another critical cross diffusion rate δc<δcsuch that cells with cross-diffusion rate δ∈(δc,δc)are stable, while, for cells with δ<δc to be stable, their degradation rate must be less than a threshold value. Hence, in some sense, cross-diffusion is harmful to enable pattern formation, while it is helpful to stabilize the cells once patterns are formed. Finally, we show that the cross diffusion plays a role in regularizing the cell aggregation phenomenon for large chemotactic coefficient. Our results provide global dynamics and insights on how the biological parameters, especially, the cross diffusion, affect pattern formations
